3. Covering and Fibration

Covering and Lifting

Definition 3.1. Let $p:E\to B$ be in $\mathsf{Top}$. A trivialization of $p$ over an open set $U\subset B$ is a homeomorphism $\phi :p^{-1}(U)\to U\times F$ over $U$, i.e. , the following diagram commutes$p$ is called locally trivial if there exists an open cover $\mathcal{U}$ of $B$ such that $p$ has a trivialization over each open $U\in \mathcal{U}$. Such $p$ is called a fiber bundle, $F$ is called the fiber and $B$ is called the base. We denote it by$$F\to E\to B,$$where there is no ambiguity from the context. If we can find a trivialization of $p$ over the whole $B$, then $E$ is homeomorphic to $F\times B$ and we say $p$ is a trivial fiber bundle.

Example 3.2. The projection map$$\begin{array}{c}\begin{array}{rcl}{\mathbb{R}}^{m+n}& \to & {\mathbb{R}}^n,\\ (x_1,\cdots ,x_n,\cdots ,x_{n+m})& \mapsto & (x_1,\cdots ,x_n)\end{array}\end{array}$$is a trivial fiber bundle with fiber ${\mathbb{R}}^m$.

Example 3.3. A real vector bundle of rank $n$ over a manifold is a fiber bundle with fiber ${\mathbb{R}}^n$.

Example 3.4. We identify $S^{2n+1}$ as the unit sphere in ${\mathbb{C}}^{n+1}$ parametrized by$$S^{2n+1}=\{z_0,z_1,\cdots ,z_n\in {\mathbb{C}}^{n+1}||z_0{|}^2+|z_1{|}^2+\cdots +|z_n{|}^2=1\}.$$There is a natural $S^1$-action on $S^{2n+1}$ given by$$e^{i\theta }:(z_0,\cdots ,z_n)\mapsto (e^{i\theta }{z}_0,\cdots ,e^{i\theta }{z}_n),e^{i\theta }\in S^1.$$This action is free, and the orbit space can be identified with the $n$-dim complex projective space $\mathbb{C}{\mathbb{P}}^n$$$S^{2n+1}/S^1\cong \mathbb{C}{\mathbb{P}}^n=({\mathbb{C}}^{n+1}-\{0\})/{\mathbb{C}}^{\ast }.$$Then the projection map $S^{2n+1}\to \mathbb{C}{\mathbb{P}}^n$ is a fiber bundle with fiber $S^1$. It is a nontrivial fact that they are not trivial fiber bundles. The case when $n=1$ gives the Hopf fibratioin$$S^1\to S^3\stackrel{p}{\to }{S}^2=\mathbb{C}{\mathbb{P}}^1$$which is particularly interesting.

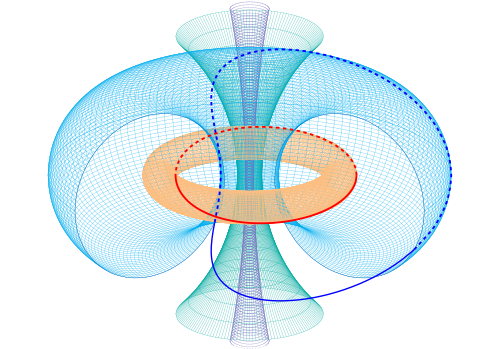
In this case, the projection sends $(z_0,z_1)\in S^3\subset {\mathbb{C}}^2$ to $z_0/z_1\in S^2=\mathbb{C}\cup \{\infty \}$. In polar coordinates, we have$$r_0^2+r_1^2=1\text{and}p(z_0,z_1)=(r_0/r_1)e^{\mathbf{i}({\theta }_0-{\theta }_1)}$$for $z_j=r_j{e}^{\mathbf{i}{\theta }_j}$. For a fixed $\rho =r_0/r_1$, we obtain a torus $T_{\rho }$ in $S^3$.

When identifying $S^3$ with the compatification of ${\mathbb{R}}^3$ (or considering the stereographic projection $S^3\to {\mathbb{R}}^3$), we can visualize (Figure 1) the foliation of ${\mathbb{R}}^3$ by these tori $T_{\rho }$, where $T_0$ degenerates to the unit circle on the $xy$-plane of ${\mathbb{R}}^3$ and $T_{\infty }$ degenerates to the $z$-axis. Each $S^1$-fiber is a slope 1 simple closed curve on one of the tori $T_{\rho }$, and the image of the projection is exactly the compatification $S^2$ of any half plane$$\{(r\cos (\phi ),r\sin (\phi ),z)\mid r>0,z\in \mathbb{R}\}$$of ${\mathbb{R}}^3$ (for a fixed $\phi \in \mathbb{R}$).

[h]

Figure 1. A visualization of Hopf fibration

Moreover, the disjoint union of any two fibers over two distinct points of $S^1$ is in fact the so called Hopf link, shown in Figure 2. Such the link is nontrivial, the Hopf fibration is not a trivial fiber bundle.

[ht]

Figure 2. Hopf link

Definition 3.5. A covering (space) is a locally trivial map $p:E\to B$ with discrete fiber $F$. A covering map which is a trivial fiber bundle is also called a trivial covering. If we would like to specify the fiber, we call it a $F$-covering. If the fiber $F$ has $n$ points, we also call it a $\mathbf{n}$-fold covering.

[h]

Figure 3. Trivialization (left) and covering (right)

Example 3.6. The map $\exp :{\mathbb{R}}^1\to S^1,t\to e^{2\pi \mathbf{i}t}$ is a $\mathbb{Z}$-covering.

[h]

Figure 4. The $\mathbb{Z}$-covering of $S^1$

If $U=S^1-\{-1\}$, then$${\exp }^{-1}(U)=\underset{n\in \mathbb{Z}}{⨆}(n-\frac{1}{2},n+\frac{1}{2}).$$

Example 3.7. The map $S^1\to S^1,e^{2\pi \mathbf{i}\theta }\mapsto e^{2\pi \mathbf{i}n\theta }$ is an $|n|$-fold covering, for any $n\in \mathbb{Z}-\{0\}$.

Example 3.8. The map $\mathbb{C}\to \mathbb{C}$, $z\mapsto z^n$, is not a covering (why?). But

•	the map ${\mathbb{C}}^{\ast }\to {\mathbb{C}}^{\ast }$, $z\mapsto z^n$, is an $|n|$-fold covering, where ${\mathbb{C}}^{\ast }=\mathbb{C}-\{0\}$and $n\in \mathbb{Z}-\{0\}$.
•	the map $\exp :\mathbb{C}\to {\mathbb{C}}^{\ast }$, $z\mapsto e^{2\pi iz}$ is a $\mathbb{Z}$-covering.

Example 3.9 (From Hatcher [??]). The figure-8has two coverings as follows (the left is a $2$-fold (or double) covering and the right is a $3$-fold covering).

[h]

The 4-regular tree is its universal cover (a covering which is simply connected), see Figure 5.

[h]

Figure 5. 4-regular tree

Example 3.10. Recall that the number of holes (genus) and the number of boundary components determine the homeomorphism type of a compact oriented topological surface. Let $S_{g,b}$ be a surface of genus $g$ and with $b$ boundary components.

•	$S_{4,0}$ admits a $7$-fold covering from $S_{22,0}$, cf. Figure 6.
•	In general, $S_{g,b}$ admits a $m$-fold covering from $S_{mg-m+1,mb}$. [h] Figure 6. A 7-covering

Example 3.11. Denote by $\mathbb{R}{\mathbb{P}}^n$ the real projective space of dimension $n$$$\mathbb{R}{\mathbb{P}}^n={\mathbb{R}}^{n+1}-\{0\}/(\underline{x}\sim t\underline{x}),\forall t\in \mathbb{R}-\{0\},\underline{x}\in {\mathbb{R}}^{n+1}-\{0\}.$$Let $S^n$ be the $n$-sphere. Then there is a natural double cover $S^n\to \mathbb{R}{\mathbb{P}}^n$.

Example 3.12 (Branched double cover). Figure 7 shows a branched double cover of a disk$$\iota :{\Sigma }_n\to D^2,\text{branching at n points.}$$

[h]

Figure 7. The Birman-Hilden double cover via the twisted surface

Namely, when deleting those $n$ (red) points (denoted by $\Delta$) from both ${\Sigma }_n$ and $D^2$, we obtain a $2$-fold covering:$$\iota :{\Sigma }_n\backslash \Delta \stackrel{2:1}{\to }{D}^2\backslash \Delta$$with a bijection $\iota :\Delta \to \Delta$ on $\Delta$.

Definition 3.13. Let $p:E\to B,f:X\to B$. A lifting of $f$ along $p$ is a map $F:X\to E$ such that $p\circ F=f$

Lemma 3.14. Let $p:E\to B$ be a covering. Let$$\begin{array}{rl}D& =\{(x,x)\in E\times E|x\in E\}\\ Z& =\{(x,y)\in E\times E|p(x)=p(y)\}.\end{array}$$Then $D\subset Z$ is both open and closed.

Proof. Exercise.

$\square$

Theorem 3.15 (Uniqueness of lifting). Let $p:E\to B$ be a covering. Let $F_0,F_1:X\to E$ be two liftings of $f:X\to B$. Suppose $X$ is connected and $F_0,F_1$ agree somewhere. Then $F_0=F_1$.

Proof. Let $D,Z$ be defined in Lemma 3.14. Consider the map $\tilde{F}=(F_0,F_1):X\to Z\subset E\times E$. By assumption, we have $\tilde{F}(X)\cap D\ne \varnothing$. Moreover, Lemma 3.14 implies that ${\tilde{F}}^{-1}(D)$ is both open and closed. Since $X$ is connected, we find ${\tilde{F}}^{-1}(D)=X$ which is equivalent to $F_0=F_1$.

$\square$

Fibration

Definition 3.16. A map $p:E\to B$ is said to have the homotopy lifting property (HLP) with respect to $X$ if for any maps $\tilde{f}:X\to E$ and $F:X\times I\to B$ such that $p\circ \tilde{f}=F{|}_{X\times \{0\}}$, there exists a lifting $\tilde{F}$ of $F$ along $p$ such that $\tilde{F}{|}_{X\times \{0\}}=\tilde{f}$, i.e., the following diagram is commutative

Definition 3.17. A map $p:E\to B$ is called a fibration (or Hurewicz fibration) if $p$ has HLP for any space.

Theorem 3.18. A covering is a fibration.

Proof. Let $p:E\to B,f:X\to B,\tilde{f}:X\to E,F:X\times I\to B$ be the data as in Definition 3.16. We only need to show the existence of ${\tilde{F}}_x$ for some neighbourhood $N_x$ of any given point $x\in X$.In fact, for any two such neighbourhoods $N_x$ and $N_y$ with $N_x\cap N_y=N_0\ne \varnothing$, we have ${\tilde{F}}_x{\mid }_{N_0}$ and ${\tilde{F}}_y{\mid }_{N_0}$ agree at some point on $\tilde{f}{\mid }_{N_0}$ and hence agree everywhere in $N_0$ by the uniqueness of lifting (Theorem 3.15). Thus $\{{\tilde{F}}_x\mid x\in X\}$ glue to give the required lifting $\tilde{F}$.

Since $I$ is compact, given $x\in X$ we can find a neighbourhood $N_x$ and a partition$$0=t_0<t_1<\cdots <t_m=1$$such that $p$ has a trivialization over open sets $U_i\supset F(N_x\times [t_i,t_{i+1}])$. Now we construct the lifting ${\tilde{F}}_x$ on $N_x\times [t_0,t_k]$, for $1\le k\le m$, by induction on $k$.

•	For $k=1$, the lifting ${\tilde{F}}_x$ on $N_x\times [t_0,t_1]$ to one of the sheets of $p^{-1}(U_1)$ is determined by $\tilde{f}{\mid }_{N_x\times \{0\}}$:
•	Assume that we have constructed ${\tilde{F}}_x$ on $N_x\times [t_0,t_k]$ for some $k$. Now, the lifting of ${\tilde{F}}_x$ on $N_x\times [t_k,t_{k+1}]$ to one of the sheets of $p^{-1}(U_k)$ is determined by $\tilde{f}{\mid }_{N_x\times \{t_k\}}$, which can be glued to the lifting on $N_x\times [t_0,t_k]$ by the uniqueness of lifting again. This finishes the inductive step.

We obtain a lifting ${\tilde{F}}_x$ of $F$ on $N_X\times I$ as required.

$\square$

Corollary 3.19. Let $p:E\to B$ be a covering. Then for any path $\gamma :I\to B$ and $e\in E$ such that $p(e)=\gamma (0)$, there exists a unique path $\tilde{\gamma }:I\to E$ which lifts $\gamma$ and $\tilde{\gamma }(0)=e$.

Proof. Apply HLP to $X=\mathrm{pt}$.

$\square$

Corollary 3.20. Let $p:E\to B$ be a covering. Then ${\Pi }_1(E)\to {\Pi }_1(B)$ is a faithful functor. In particular, the induced map ${\pi }_1(E,e)\to {\pi }_1(B,p(e))$ is injective.

Proof. Let ${\tilde{\gamma }}_i:I\to E$ be two paths and $[{\tilde{\gamma }}_i]\in {\mathrm{Hom}}_{{\Pi }_1(E)}(e_1,e_2)$. Let ${\gamma }_i=p\circ {\tilde{\gamma }}_i$. Suppose $[{\gamma }_1]=[{\gamma }_2]$ and we need to show that $[{\tilde{\gamma }}_1]=[{\tilde{\gamma }}_2]$.

Let $F:{\gamma }_1\simeq {\gamma }_2$ be a homotopy. Consider the following commutative diagram with the lifting $\tilde{F}$ by HLPThen the uniqueness of lifting implies $\tilde{F}{\mid }_{I\times \{1\}}={\tilde{\gamma }}_2$. Thus, $\tilde{F}:{\tilde{\gamma }}_1\simeq {\tilde{\gamma }}_2$.

$\square$

Transport functor

Let $p:E\to B$ be a covering. Let $\gamma :I\to B$ be a path in $B$ from $b_0$ to $b_1$. It defines a map$$\begin{array}{rl}{T}_{\gamma }:p^{-1}(b_0)& \to p^{-1}(b_1)\\ e_0& \mapsto e_1=\tilde{\gamma }(1)\end{array}$$where $\tilde{\gamma }$ is a lift of $\gamma$ with initial condition $\tilde{\gamma }(0)=e_0$.

[h]

Figure 8. The transportation

Assume $[{\gamma }_1]=[{\gamma }_2]$ in $B$. HLP implies that $T_{{\gamma }_1}=T_{{\gamma }_2}$. We find a well-defined map:$$\begin{array}{rl}T:{\mathrm{Hom}}_{{\Pi }_1(B)}(b_1,b_2)& \to {\mathrm{Hom}}_{\mathsf{Set}}(p^{-1}(b_1),p^{-1}(b_2))\\ [\gamma ]& \mapsto T_{[\gamma ]}.\end{array}$$

This leads to the following definition (check the functor property!).

Definition 3.21. The following data$$\begin{array}{rl}T:{\Pi }_1(B)& \to \mathsf{Set}\\ b& \to p^{-1}(b)\\ [\gamma ]& \mapsto T_{[\gamma ]}\end{array}$$define a functor, called the transport functor. In particular, we have a well-defined map$${\pi }_1(B,b)={\mathrm{Aut}}_{{\Pi }_q(B)}(b)\to {\mathrm{Aut}}_{\mathsf{Set}}(p^{-1}(b)).$$Here ${\mathrm{Aut}}_{\mathsf{Set}}(S)$ consists of all set isomorphisms in ${\mathrm{Hom}}_{\mathsf{Set}}(S,S)$ for $S\in \mathrm{Obj}(\mathsf{Set})$.

Example 3.22. Consider the covering map$$\mathbb{Z}\to {\mathbb{R}}^1\stackrel{\exp }{\to }{S}^1.$$Consider the following path representing an element of ${\pi }_1(S^1)$$${\gamma }_n:I\to S^1,t\to \exp (nt)=e^{2\pi int},n\in \mathbb{Z}.$$

Start with any point $m\in \mathbb{Z}$ in the fiber, ${\gamma }_n$ lifts to a map to ${\mathbb{R}}^1$$${\tilde{\gamma }}_n:I\to R^1,t\mapsto m+nt.$$We find $T_{[{\gamma }_n]}(m)=\tilde{\gamma }(1)=m+n$. Therefore $T_{[{\gamma }_n]}\in {\mathrm{Aut}}_{\mathsf{Set}}(\mathbb{Z})$ is$$T_{[{\gamma }_n]}:\mathbb{Z}\to \mathbb{Z},m\mapsto m+n.$$

Proposition 3.23. Let $p:E\to B$ be a covering, $E$ be path connected. Let $e\in E,b=p(e)\in B$. Then the action of ${\pi }_1(B,b)$ on $p^{-1}(b)$ is transitive, whose stabilizer ${\mathrm{Stab}}_e({\pi }_1(B,b))$ at $e$ is ${\pi }_1(E,e)$. In other words,$$p^{-1}(b)\cong {\pi }_1(B,b)/{\pi }_1(E,e)$$as a coset space, i.e. we have the following short exact sequence$$\begin{array}{rl}1\to {\pi }_1(E,e)\to {\pi }_1(B,b)& \stackrel{{\partial }_e}{\to }{p}^{-1}(b)\to 1.\\ [\gamma ]& \mapsto T_{\gamma }(e)\end{array}$$

Proof. For any point $e^{\prime }\in p^{-1}(b)$, let $\tilde{\gamma }:e\to e^{\prime }$ be a path in $E$ and $\gamma =p\circ \tilde{\gamma }$. Then $e^{\prime }={\partial }_e([\gamma ])$. This shows the surjectivity of ${\partial }_e$.

HLP implies that $p_{\ast }:{\pi }_1(E,e)\to {\pi }_1(B,b)$ is injective and we can view ${\pi }_1(E,e)$ as a subgroup of ${\pi }_1(B,b)$. By definition, for $\tilde{\gamma }\in {\pi }_1(E,e)$, we have ${\partial }_e([p\circ \tilde{\gamma }])=\tilde{\gamma }(1)=e$, i.e. ${\pi }_1(E,e)\subset {\mathrm{Stab}}_e({\pi }_1(B,b))$. On the other hand, if $T_{\gamma }(e)=e$, then the lift $\tilde{\gamma }$ of $\gamma$ is a loop, i.e. $\tilde{\gamma }\in {\pi }_1(E,e)$. Therefore, ${\pi }_1(E,e)\supset {\mathrm{Stab}}_e({\pi }_1(B,b))$. This implies ${\pi }_1(E,e)={\mathrm{Stab}}_e({\pi }_1(B,b))$, which finishes the proof.

$\square$

Lifting Criterion

Theorem 3.24 (Lifting Criterion). Let $p:E\to B$ be a covering. Consider a continuous map $f:X\to B$, where $X$ is path connected and locally path connected. Let $e_0\in E,x_0\in X$ such that $f(x_0)=p(e_0)$. Then there exists a lift $F$ of $f$ with $F(x_0)=e_0$ if and only if$$f_{\ast }({\pi }_1(X,x_0))\subset p_{\ast }({\pi }_1(E,e_0)).$$

Proof. If such $F$ exists, then$$f_{\ast }({\pi }_1(X,x_0))=p_{\ast }(F_{\ast }({\pi }_1(X,x_0)))\subset p_{\ast }({\pi }_1(E,e_0)).$$Conversely, let$$\tilde{E}=\{(x,e)\in X\times E|f(x)=p(e)\}\subset X\times E$$and consider the following commutative diagramThe projection $\tilde{p}$ is also a covering. We have an induced commutative diagram of functorswhich induces natural group homomorphisms$${\pi }_1(X,x_0)\stackrel{f_{\ast }}{\to }{\pi }_1(B,b_0)\to \mathrm{Aut}(p^{-1}(b_0))=\mathrm{Aut}({\tilde{p}}^{-1}(x_0)),b_0=f(x_0)=p(e).$$Let ${\tilde{e}}_0=(x_0,e_0)\in \tilde{E}$. The condition $f_{\ast }({\pi }_1(X,x_0))\subset p_{\ast }({\pi }_1(E,e_0))$ says that ${\pi }_1(X,x_0)$ stabilizes ${\tilde{e}}_0$. By Proposition 3.23, this implies we have a group isomorphism$${\tilde{p}}_{\ast }:{\pi }_1(\tilde{E},{\tilde{e}}_0)\cong {\pi }_1(X,x_0).$$Since $X$ is locally path connected, $\tilde{E}$ is also locally path connected. Then path connected components and connected components of $\tilde{E}$ coincide. Let $\tilde{X}$ be the (path) connected component of $\tilde{E}$ containing ${\tilde{e}}_0$, then ${\pi }_1(\tilde{E},\tilde{e})\cong {\pi }_1(X,x_0)$ implies that $\tilde{p}:\tilde{X}\to X$ is a covering with fiber a single point, hence a homeomorphism. Its inverse defines a continuous map $X\to \tilde{E}$ whose composition with $\tilde{E}\to E$ gives $F$.

$\square$

$G$-principal covering

Definition 3.25. Let $G$ be a discrete group. A continuous action $G\times X\to X$ is called properly discontinuous if for any $x\in X$, there exists an open neighborhood $U$ of $x$ such that$$\begin{array}{c}g(U)\cap U=\varnothing ,\forall g\ne 1\in G.\end{array}$$We define the orbit space $X/G=X/\sim$ where $x\sim g(x)$ for any $x\in X,g\in G$.

Proposition 3.26. Assume $G$ acts properly discontinuously on $X$, then the quotient map $X\to X/G$ is a covering.

Proof. For any $x\in X$, let $U$ be the neighbourhood satisfying $g(U)\cap U=\varnothing ,\forall g\ne 1\in G$. Then$$p^{-1}\left(p(U)\right)=\underset{g\in G}{⨆}gU$$is a disjoint union of open sets. Thus, $p$ is locally trivial with discrete fiber $G$, hence a covering.

$\square$

Definition 3.27. A left (right) $\mathbf{G}$-principal covering is a covering $p:E\to B$ with a left (right) properly discontinuous $G$-action on $E$ over $B$such that the induced map $E/G\to B$ is a homeomorphism.

Example 3.28. $\exp :{\mathbb{R}}^1\to S^1$ is a $\mathbb{Z}$-principal covering for the action $n:t\to t+n,\forall n\in \mathbb{Z}$.

Example 3.29. $S^n\to \mathbb{R}{P}^n\cong S^n/{\mathbb{Z}}_2$ is a ${\mathbb{Z}}_2$-principal covering.

Proposition 3.30. Let $p:E\to B$ be a $G$-principal covering. Then the transport is $G$-equivariant, i.e.,$$T_{[\gamma ]}\circ g=g\circ T_{[\gamma ]},\forall g\in G,\gamma \text{a path in}B.$$

Proof. Let $\gamma :b_0\to b_1$ and $e_0\in p^{-1}(b_0)$. Then $\tilde{\gamma }:e_0\to e_1=T_{[\gamma ]}(e_0)$ for some $e_1\in p^{-1}(b_1)$. If we apply the transformation $g$ to the path $\tilde{\gamma }$, we find another lift of $\gamma$ but with endpoints $g(e_0)$ and $g(e_1)$. Therefore$$T_{[\gamma ]}(g(e_0))=g(e_1).$$It follows that $T_{[\gamma ]}(g(e_0))=g(e_1)=g(T_{[\gamma ]}(e_0))$. This proves the proposition.

[h]

Figure 9. Transport commutes with $G$-action

$\square$

Theorem 3.31. Let $p:E\to B$ be a $G$-principal covering, $E$ path connected, $e\in E,b=p(e)$. Then we have an exact sequence of groups$$1\to {\pi }_1(E,e)\to {\pi }_1(B,b)\to G\to 1.$$In other words, ${\pi }_1(E,e)$ is a normal subgroup of ${\pi }_1(B,b)$ and $G={\pi }_1(B,b)/{\pi }_1(E,e)$.

Proof. Let $F=p^{-1}(b)$. The previous proposition implies that ${\pi }_1(B,b)$-action and $G$-action on $F$ commute. It induces a ${\pi }_1(B,b)\times G$-action on $F$. Consider its stabilizer at $e$ and two projections${\mathrm{pr}}_1$ is an isomorphism and ${\mathrm{pr}}_2$ is an epimorphism with $\ker ({\mathrm{pr}}_2)={\text{Stab}}_e({\pi }_1(B,b))={\pi }_1(E,e)$.

$\square$

Universal cover

Definition 3.32. The universal cover of $B$ is a covering map $p:E\to B$ with $E$ simply connected.

The universal cover is unique (if exists) up to homeomorphism. This follows from the lifting criterion and the unique lifting property of covering maps. We left it as an exercise to readers.

Definition 3.33. A space is semi-locally simply connected if for any $x_0\in X$, there is a neighbourhood $U_0$ such that the image of the map $i_{\ast }:{\pi }_1(U_0,x_0)\to {\pi }_1(X,x_0)$ is trivial.

We recall the following theorem from point-set topology.

Theorem 3.34 (Existence of the universal cover). Assume $B$ is path connected and locally path connected. Then universal cover of $B$ exists if and only if $B$ is semi-locally simply connected.

The next theorem is a direct consequence of Theorem 3.31.

Theorem 3.35. Assume $p:E\to B$ is a universal cover. Then the fiber $p^{-1}(b)$ can be identified with ${\pi }_1(B,b)$ as a set. If $p$ is a $G$-covering, then we have a group isomorphism ${\pi }_1(B)=G.$

Example 3.36. Apply this theorem to the covering $\exp :{\mathbb{R}}^1\to S^1$, we find a group isomorphism$$\mathrm{deg}:{\pi }_1(S^1)\stackrel{\cong }{\to }\mathbb{Z}$$which is called the degree map. An example of degree $n$ map is$$S^1\to S^1,e^{i\theta }\mapsto e^{in\theta }.$$

Classical applications

Definition 3.37. Let $i:A\subset X$ be an inclusion. A continuous map $r:X\to A$ is called a retraction if $r\circ i=1_A$. It is called a deformation retraction if furthermore we have a homotopy $i\circ r\simeq 1_X\mathrm{rel}A$. We say $A$ is a (deformation) retract of $X$ if such a (deformation) retraction exists.

Proposition 3.38. If $i:A\subset X$ is a retract, then $i_{\ast }:{\pi }_1(A)\to {\pi }_1(X)$ is injective.

Proof. Let $r:X\to A$ such that $r\circ i=1_A$. Then the composition$${\pi }_1(A)\stackrel{i_{\ast }}{\to }{\pi }_1(X)\stackrel{r_{\ast }}{\to }{\pi }_1(A)$$is the identity. It follows that $i_{\ast }:{\pi }_1(A)\to {\pi }_1(X)$ is injective.

$\square$

Corollary 3.39. Let $D^2$ be the unit disk in ${\mathbb{R}}^2$. Then its boundary $S^1$ is not a retract of $D^2$.

Proof. Since $D^2$ is contractible, we have ${\pi }_1(D^2)=1$. But ${\pi }_1(S^1)=\mathbb{Z}$. Then the corollary follows from the proposition above.

$\square$

Theorem 3.40 (Brouwer fixed point Theorem). Let $f:D^2\to D^2$. Then there exists $x\in D^2$ such that $f(x)=x$.

Proof. Assume $f$ has no fixed point. Let $l_x$ be the ray starting from $f(x)$ pointing toward $x$. Then$$D^2\to S^1,x\mapsto l_x\cap \partial D^2$$is a retraction of $\partial D^2=S^1\subset D^2$. Contradiction.

$\square$

Theorem 3.41 (Fundamental Theorem of Algebra). Let $f(x)=x^n+c_1{x}^{n-1}+\cdots +c_n$ be a polynomial with $c_i\in \mathbb{C},n>0$. Then there exists $a\in \mathbb{C}$ such that $f(a)=0$.

Proof. Assume $f$ has no root in $\mathbb{C}$. Define a homotopy of maps from $S^1$ to $S^1$$$F:S^1\times I\to S^1,F(e^{i\theta },t)=\frac{f(\tan (\frac{\pi t}{2})e^{i\theta })}{\left|f(\tan (\frac{\pi t}{2})e^{i\theta })\right|}.$$By construction, $\mathrm{deg}(F{|}_{S^1\times 0})=0$ and $\mathrm{deg}(F{|}_{S^1\times 1})=n$. But they are homotopic hence representing the same element in ${\pi }_1(S^1)$. Contradiction.

$\square$

Proposition 3.42 (Antipode). Let $f:S^1\to S^1$ be an antipode-preserving map, i.e. $f(-u)=-f(u)$. Then $\mathrm{deg}(f)$ is odd. In particular, $f$ is NOT null homotopic.

Proof. Let $F:{\mathbb{R}}^1\to {\mathbb{R}}^1$ be a lifting of $f\circ \exp :{\mathbb{R}}^1\to S^1$ for the covering map $\exp :{\mathbb{R}}^1\to S^1$. Then$$\begin{array}{r}F(x+1)=F(x)+\mathrm{deg}(f).\end{array}$$Since $f$ is antipode-preserving, $F(x+1/2)=F(x)+m$ for some $m\in \mathbb{Z}+1/2$. So $F(x+1)=F(x)+2m$ which implies $\mathrm{deg}(f)=2m$ is odd.

$\square$

Example 3.43. Let $\sigma :S^1\to S^1$ be the antipode map defined by $\sigma (u)=-u$. Then $\mathrm{deg}(\sigma )=1$.

Theorem 3.44 (Borsuk-Ulam). Let $f:S^2\to {\mathbb{R}}^2$. Then there exists $x\in S^2$ such that $f(x)=f(-x)$.

Proof. Assume $f(x)\ne f(-x),\forall x\in S^2$. Define$$\rho :S^2\to S^1,\rho (x)=\frac{f(x)-f(-x)}{\left|f(x)-f(-x)\right|}.$$Let $D^2$ be the upper hemi-sphere of $S^2$. It defines a homotopy between constant map and $\rho {|}_{\partial D^2}:S^1\to S^1$, hence $\mathrm{deg}(\rho {|}_{\partial D^2})=0$. On the other hand, $\rho {|}_{\partial D^2}$ is antipode-preserving: $\rho {|}_{\partial D^2}(-x)=-\rho {|}_{\partial D^2}(x)$, hence $\mathrm{deg}(\rho {|}_{\partial D^2})$ is odd. Contradiction.

$\square$

Theorem 3.45 (Ham Sandwich Theorem). Let $A_1,A_2$ be two bounded regions of positive areas in ${\mathbb{R}}^2$. Then there exists a line which cuts each $A_i$ into half of equal areas.

[ht]

Proof. Let us put $A_1,A_2\subset {\mathbb{R}}^2\times \{1\}\subset {\mathbb{R}}^3$, cf. the orange triangle and green quadrilateral Figure 9 as illustration.

Given $u\in S^2$, let $P_u$ be the plane passing the origin and perpendicular to the unit vector $u$. Let $A_i(u)=\{p\in A_i|p\cdot u\le 0\}.$ Define the continuous map$$f=(f_1,f_2):S^2\to {\mathbb{R}}^2,f_i(u)=\text{Area}(A_i(u)).$$By Borsuk-Ulam, $\exists u$ such that $f(u)=f(-u)$. The intersection ${\mathbb{R}}^2\times \{1\}\cap P_u$ gives the required line since$$f(u)=f(-u)⟺f_i(u)=\frac{1}{2}\text{Area}(A_i).$$

$\square$

Classification of covering

Definition 3.46. We define the category $\text{Cov}(B)$ of coverings of $B$ where

•	an object is a covering map $p:E\to B$;
•	a morphism between two coverings $p_1:E_1\to B$ and $p_2:E_2\to B$ is a map $f:E_1\to E_2$ such that the following diagram is commutative

Definition 3.47. Let $B$ be connected. We define ${\text{Cov}}_0(B)\subset \text{Cov}(B)$ to be the subcategory whose objects consist of coverings of $B$ which are connected spaces.

Proposition 3.48. Let $B$ be connected and locally path connected. Then any morphism in ${\text{Cov}}_0(B)$ is a covering map.

Proof. Exercise.

$\square$

Definition 3.49. We define the category $G-\mathsf{Set}$, where

•	an object is a set $S$ with $G$-action and
•	morphisms are $G$-equivariant set maps, i.e. $f:S_1\to S_2$ such that $f\circ g=g\circ f$, for any $g\in G$.

Given a covering $p:E\to B$, $b\in B$, the transport functor shows$$p^{-1}(b)\in {\pi }_1(B,b)-\mathsf{Set}.$$

Lemma 3.50. Let $B$ be path connected. Then ${\pi }_1(B,b)$ acts transitively on $p^{-1}(b)$ if and only if $E$ is path connected.

Proof. The "if" part follows from Proposition 3.23. We prove the "only if" part.

Let us fix a point $e_0\in p^{-1}(b)$. Assume ${\pi }_1(B,b)$ acts transitively on $p^{-1}(b)$. This implies that any point in $p^{-1}(b)$ is connected to $e_0$ by a path. Given any point $e\in E$, let $\gamma$ be a path in $B$ that connects $p(e)$ to $b$. The transport functor $T_{[\gamma ]}$ gives a path connecting $e$ to some point in $p^{-1}(b)$. This further implies that $e$ is path connected to $e_0$. This proves the "only if" part.

[h]

Figure 10. Transitivity v.s. path connectedness

$\square$

Corollary 3.51. Let $B$ be path connected, $p:E\to B$ be a covering. Then there is a one-to-one correspondence between path connected components of $E$ and ${\pi }_1(B,b)$-orbits in $p^{-1}(b)$.

Proof. Exercise.

$\square$

Proposition 3.52. Assume $B$ is path connected and locally path connected. Let $p_1,p_2\in \text{Cov}(B)$. Then$${\mathrm{Hom}}_{\text{Cov}(B)}(p_1,p_2)\cong {\mathrm{Hom}}_{{\pi }_1(B,b)-\mathsf{Set}}(p_1^{-1}(b),p_2^{-1}(b))$$for any $b\in B$.

Proof. Let $f\in {\mathrm{Hom}}_{\text{Cov}(B)}(p_1,p_2)$, i.e.By restricting $f$ to the fiber $p^{-1}(b)$, it induces a map$$f_b:p_1^{-1}(b)\to p_2^{-1}(b).$$By the same argument as in Proposition 3.30, we find $f_b$ is ${\pi }_1(B,b)$-equivariant. Thus we obtain a map$$\begin{array}{rl}\Phi :{\mathrm{Hom}}_{\text{Cov}(B)}(p_1,p_2)& \to {\mathrm{Hom}}_{{\pi }_1(B,b)-\mathsf{Set}}(p_1^{-1}(b),p_2^{-1}(b))\\ f& \to f_b\end{array}$$

The injectivity of $\Phi$ follows from the uniqueness of the lifting.

To prove surjectivity, we can assume $E_1$ is path connected, thus ${\pi }_1(B,b)$ acts transitively on $p_1^{-1}(b)$ (Corollary 3.51). Given $f_b:p_1^{-1}(b)\to p_2^{-1}(b)$, let us fix two points $e_i\in p_i^{-1}(b)$ such that $f(e_1)=e_2$. The ${\pi }_1(B,b)$-equivariance of $f_b$ gives rise to the homomorphism$$\begin{array}{lll}& {\mathrm{Stab}}_{e_1}({\pi }_1(B,b))⟶& {\mathrm{Stab}}_{e_2}({\pi }_1(B,b))\\ & ={\pi }_1(E_1,e_1)& ={\pi }_1(E_2,e_2).\end{array}$$By Lifting Criterion (Theorem 3.24), we obtain a morphism $f:E_1\to E_2$ as required.

$\square$

Theorem 3.53. Assume $B$ is path connected, locally path connected and semi-locally simply connected. $b\in B$. Then there exists an equivalence of categories$$\text{Cov}(B)\simeq {\pi }_1(B,b)-\mathsf{Set}.$$

Proof. Let us denote ${\pi }_1={\pi }_1(B,b)$. Let $\tilde{p}:\tilde{B}\to B$ be a fixed universal cover of $B$ and $\tilde{b}\in {\pi }^{-1}(b)$ chosen.

We will define the following functorsLet $p:E\to B$ be a covering, we define$$F(p):=p^{-1}(b).$$Let $S\in {\pi }_1-\mathsf{Set}$, we define$$G(S):=\tilde{B}{\times }_{{\pi }_1}S=\tilde{B}\times S/\sim ,$$where $(e\cdot g,s)\sim (e,g\cdot s)$ for any $e\in \tilde{B},s\in S,g\in {\pi }_1$. Note that here $e\cdot g$ represents the (right) ${\pi }_1$-action on $\tilde{B}$. Then we have natural isomorphisms$$F\circ G\stackrel{\eta }{\simeq }1,G\circ F\stackrel{\tau }{\simeq }1.$$Here $\eta$ is the natural isomorphism$${\eta }_S\in {\mathrm{Hom}}_{{\pi }_1-\mathsf{Set}}(F\circ G(S),S),{\eta }_S(e,s)=g\cdot s\text{if}e=\tilde{b}\cdot g.$$$\tau$ is the natural isomorphism$${\tau }_p\in {\mathrm{Hom}}_{\text{Cov}(B)}(p^{\prime },p)\cong {\mathrm{Hom}}_{{\pi }_1-\mathsf{Set}}(p^{-1}(b),p^{-1}(b)),p^{\prime }=G\circ F(p):\tilde{B}{\times }_{{\pi }_1}{p}^{-1}(b)\to B,$$which is determined by the identity map in ${\mathrm{Hom}}_{{\pi }_1-\mathsf{Set}}(p^{-1}(b),p^{-1}(b))$.

$\square$

Definition 3.54. Let $B$ be path connected and $p:E\to B$ be a connected covering. A deck transformation (or covering transformation) of $p$ is a homeomorphism $f:E\to E$ such that $p\circ f=p$.Let $\mathrm{Aut}(p)$ denote the group of deck transformations.

Note that $\mathrm{Aut}(p)$ acts freely on $E$ by the Uniqueness of Lifting.

Proposition 3.55. Let $B$ be path connected and $p:E\to B$ be a connected covering. Then $\mathrm{Aut}(p)$ acts properly discontinuously on $E$.

Proof. Exercise.

$\square$

Corollary 3.56. Assume $B$ is path connected, locally path connected. Let $p:E\to B$ be a connected covering, $e\in E,b=p(e)\in B$, $G={\pi }_1(B,b),H={\pi }_1(E,e)$. Then$$\mathrm{Aut}(p)\cong N_G(H)/H$$where$$N_G(H):=\{r\in G\mid rH{r}^{-1}=H\}$$is the normalizer of $H$ in $G$.

Proof. By Proposition 3.23, Lemma 3.50 and Theorem 3.53$$\mathrm{Aut}(p)\cong {\mathrm{Hom}}_{G-\mathsf{Set}}(G/H,G/H)=N_G(H)/H.$$

$\square$

Definition 3.57. We define the orbit category $Orb(G)$:

•	objects consist of (left) coset $G/H$, where $H$ is a subgroup of $G$;
•	morphisms are $G$-equivariant maps: $G/H_1\to G/H_2$.

Note $G/H_1$ and $G/H_2$ are isomorphic in $Orb(G)$ if and only if $H_1$ and $H_2$ are conjugate subgroups of $G$.

If we restrict Theorem 3.53 to connected coverings, we find

Theorem 3.58. Assume $B$ is path connected, locally path connected and semi-locally simply connected. $b\in B$. Then there exists an equivalence of categories$${\text{Cov}}_0(B)\simeq Orb({\pi }_1(B,b)).$$

The universal cover $\tilde{B}\to B$ corresponds to the orbit ${\pi }_1(B,b)$. For the orbit ${\pi }_1(B,b)/H$, it corresponds to$$E=\tilde{B}/H\to B.$$This can be illustrated by the following correspondence

A more intrinsic formulation is as follows. Given a covering $p:E\to B$, we obtain a transport functor$$T_p:{\Pi }_1(B)\to \mathsf{Set}.$$Given a commutative diagramwe find a natural transformation$$\begin{array}{r}\tau :T_{p_1}⟹T_{p_2},\tau =\{f:p_1^{-1}(b)\to p_2^{-1}(b)|b\in B\}.\end{array}$$

The above structure can be summarized by a functor$$T:\text{Cov}(B)\to \mathsf{Fun}({\Pi }_1(B),\mathsf{Set}).$$

Theorem 3.59. Assume $B$ is path connected, locally path connected and semi-locally simply connected. Then $$T:\text{Cov}(B)\to \mathsf{Fun}({\Pi }_1(B),\mathsf{Set})$$is an equivalence of categories.