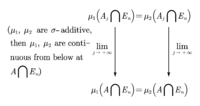4. Caratheodory theorem
(待修改! ) Given a semi-algebra over and an additive function , we prove that there exist a unique extension which is additive in the last note. In this note, our goal is that prove the following fact
##### Step 1
Definition
Given an algebra over and a function , we define Definition
Given where contain the empty set and we say a function is an outer measure on if
- - and , - and
Claim
is an outer measure.
- Take , then
Therefore since the codomain of is nonnegative.
- Let and , it is clear that . Set and . It is clear that , then
- Take a sequence , we assume that for all (note that if for some , then the inequality holds trivially), . Fix , there always exist a sequence such that
Also, since . Then holds for any . So .
##### Step 2
We define a family of subsets , if satisfy We claim that
-
- is a -algebra
Observation since .
So, if we want to prove that where , it sufficient to show that for . Not loss the generality, we may assume that since the inequality holds trivially while . Given , we can find such that
We obtain since and . Similarly, we obtain Therefore, holds for any . So we have and hence . It is clear that since .
Assume that , then (by the definition of ) we have holds for every . By the symmetry of and , .
Given , we need to show that .
Remark The property is equal to .
First, we show that , . It sufficient to show the in-equality holds for . Since , , we have and Take the second formula into the first one we obtain π∗(E)=π∗(E⋂A)+π∗((E\A)⋂B)+π∗((E\A)\B)=π∗(E⋂A)+π∗((E\A)⋂B)+π∗(E\(A⋃B))=π∗(E⋂A)+π∗((E\A)⋂B)+π∗(E⋂(A⋃B)c) Then it sufficient to show that By the property of , it sufficient to show that Actually,
Next, we show that is closed by countable union (i.e. ).
It sufficient to show the following inequality But we know that is closed by finite union We take F1F2Fn=A1=A2\A1⋮=An\(A1⋃⋯⋃An−1) Then and it is clear that for any and where and Fj(M is closed by ( )c and finite union)=Aj\(A1⋃⋯⋃Aj−1)=Aj⋂(A1⋃⋯⋃Aj−1)c∈M Therefore, Claim By induction
, the equality holds trivially
Assume that the equality holds for
for , we have
We rewrite the inequality to
Since the inequality is correct for any , the following inequality can be hold. Therefore, It completed the proof that is a -algebra.
We now know that
- - is a -algebra
Then we obtain the fact .
##### Step 3
The restriction is a extension of which define on the algebra and is -additive.
- where
Set , then .
- where and
We take F1F2Fn=E1=E2\E1⋮=En\(E1⋃⋯⋃En−1) It is clear that and , for any and where . Therefore, . We conclude This inequality holds for all covering of , so
- is -additive
Take such that for all and where . we conclude that and Then we can take to be , and we get the inequality Consequently, is an extension of and is -additive.
##### Step 4
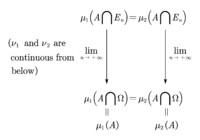
**Goal** Given two functions , such that . If is -finite with respect to and , then .
Remark Given a set , we say that is -finite with respect to if for any increasing converges sequence , for all .
Definition
Given a set and , we say that is a monotone class if
- is an increasing converges sequence - is an decreasing converges sequence
Assertion If is a family of monotone classes where is an index set. Then is a monotone class (is easy to see).
Given a class , we define and is the smallest monotone class which contain .
Remark since is a monotone class itself ,so this definition make sense.
<a id="lemma">Lemma</a> Given an algebra , we have the equality Given an increasing converges sequence and fix , we define
Take an element , since .
is a monotone class
Given an increasing converges sequence, then
since , then we have .
Similarly, Given an decreasing converges sequence, then
So is a monotone class.
Therefore, . By using [the lemma](#lemma) we have , but, by the construction of , . Consequently, Given , then , we have the following diagram